So far, we have considered various forms of energy such as heat Q, work W, and total energy E individually, and no attempt is made to relate them to each other during a process. The first law of thermodynamics, also known as the conservation of energy principle, provides a sound basis for studying the relationships among the various forms of energy and energy interactions.
Daftar Isi
Based on experimental observations, the first law of thermodynamics states that energy can be neither created nor destroyed during a process; it can only change forms. Therefore, every bit of energy should be accounted for during a process.

destroyed, it can only change forms
We all know that a rock at some elevation possesses some potential energy, and part of this potential energy is converted to kinetic energy as the rock falls. Experimental data show that the decrease in potential energy (mgΔz) exactly equals the increase in kinetic energy:
E=\frac{1}{2}m(v_2^2-v_1^2)when the air resistance is negligible, thus confirming the conservation of energy principle for mechanical energy.
Consider a system undergoing a series of adiabatic processes from a specified state 1 to another specified state 2. Being adiabatic, these processes obviously cannot involve any heat transfer, but they may involve several kinds of work interactions. Careful measurements during these experiments indicate the following: For all adiabatic processes between two specified states of a closed system, the net work done is the same regardless of the nature of the closed system and the details of the process. Considering that there are an infinite number of ways to perform work interactions under adiabatic conditions, this statement appears to be very powerful, with a potential for far-reaching implications. This statement, which is largely based on the experiments of Joule in the first half of the nineteenth century, cannot be drawn from any other known physical principle and is recognized as a fundamental principle. This principle is called the first law of thermodynamics or just the first law.
A major consequence of the first law is the existence and the definition of the property total energy E. Considering that the net work is the same for all adiabatic processes of a closed system between two specified states, the value of the net work must depend on the end states of the system only, and thus it must correspond to a change in a property of the system. This property is the total energy. Note that the first law makes no reference to the value of the total energy of a closed system at a state. It simply states that the change in the total energy during an adiabatic process must be equal to the net work done. Therefore, any convenient arbitrary value can be assigned to total energy at a specified state to serve as a reference point.
Implicit in the first law statement is the conservation of energy. Although the essence of the first law is the existence of the property total energy, the first law is often viewed as a statement of the conservation of energy principle. Next we develop the first law or the conservation of energy relation with the help of some familiar examples using intuitive arguments.

First, we consider some processes that involve heat transfer but no work interactions. The potato baked in the oven is a good example for this case. As a result of heat transfer to the potato, the energy of the potato will increase.
If we disregard any mass transfer (moisture loss from the potato), the increase in the total energy of the potato becomes equal to the amount of heat transfer. That is, if 5 kJ of heat is transferred to the potato, the energy increase of the potato will also be 5 kJ.

As another example, consider the heating of water in a pan on top of a range. If 15 kJ of heat is transferred to the water from the heating element and 3 kJ of it is lost from the water to the surrounding air, the increase in energy of the water will be equal to the net heat transfer to water, which is 12 kJ.
Now consider a well-insulated (i.e., adiabatic) room heated by an electric heater as our system. As a result of electrical work done, the energy of the system will increase. Since the system is adiabatic and cannot have any heat transfer to or from the surroundings (Q=0), the conservation of energy principle dictates that the electrical work done on the system must equal the increase in energy of the system.


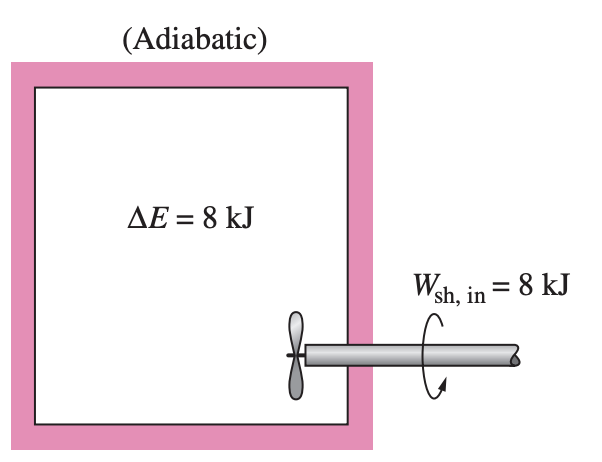
Next, let us replace the electric heater with a paddle wheel. As a result of the stirring process, the energy of the system will increase. Again, since there is no heat interaction between the system and its surroundings (Q=0), the shaft work done on the system must show up as an increase in the energy of the system.
Many of you have probably noticed that the temperature of air rises when it is compressed. This is because energy is transferred to the air in the form of boundary work. In the absence of any heat transfer (Q=0), the entire boundary work will be stored in the air as part of its total energy. The conservation of energy principle again requires that the increase in the energy of the system be equal to the boundary work done on the system.


We can extend these discussions to systems that involve various heat and work interactions simultaneously. For example, if a system gains 12 kJ of heat during a process while 6 kJ of work is done on it, the increase in the energy of the system during that process is 18 kJ. That is, the change in the energy of a system during a process is simply equal to the net energy transfer to (or from) the system.
Energy Balance
In the light of the preceding discussions, the conservation of energy principle can be expressed as follows: The net change (increase or decrease) in the total energy of the system during a process is equal to the difference between the total energy entering and the total energy leaving the system
during that process.
Ein-Eout=ΔEsystem
This relation is often referred to as the energy balance and is applicable to any kind of system undergoing any kind of process. The successful use of this relation to solve engineering problems depends on understanding the various forms of energy and recognizing the forms of energy transfer.
Energy Change of a System
The determination of the energy change of a system during a process involves the evaluation of the energy of the system at the beginning and at the end of the process, and taking their difference. That is,
Energy change= Energy at final state – Energy at initial state
or
ΔEsystem= Efinal-Einitial=E2-E1
Note that energy is a property, and the value of a property does not change unless the state of the system changes. Therefore, the energy change of a system is zero if the state of the system does not change during the process. Also, energy can exist in numerous forms such as internal (sensible, latent, chemical, and nuclear), kinetic, potential, electric, and magnetic, and their sum constitutes the total energy E of a system. In the absence of electric, magnetic, and surface tension effects (i.e., for simple compressible systems), the change in the total energy of a system during a process is the sum of the changes in its internal, kinetic, and potential energies and can be expressed as :
ΔE=ΔU+ΔKE+ΔPE
where
ΔU=m(u_2-u_1)
ΔE_K=\frac{1}{2}m(v_2^1-v_1^2)ΔE_P=mg(z_2-z_1)
When the initial and final states are specified, the values of the specific internal energies u1 and u2 can be determined directly from the property tables or thermodynamic property relations.
Most systems encountered in practice are stationary, that is, they do not involve any changes in their velocity or elevation during a process (Fig. 2–44). Thus, for stationary systems, the changes in kinetic and potential energies are zero (that is, KE PE 0), and the total energy change relation in Eq. 2–33 reduces to E U for such systems. Also, the energy of a system during a process will change even if only one form of its energy changes while the other forms of energy remain unchanged.
Leave a Reply
You must be logged in to post a comment.